NCERT Solutions for Maths Class 8 Chapter 3 Exercise 3.3 – FREE PDF Download
Class 8 Maths Exercise 3.3 Solutions
Class 8 Maths Exercise 3.3 in Hindi
Class 8 Maths Book Download in PDF
Class 8 Maths Chapter 3 Solutions
Class 8 Maths NCERT Solutions
Class 8 All Subjects Solutions
NCERT Solutions for Class 8 Maths Chapter 3 Exercise 3.3 in Hindi and English Medium modified and updated for academic year 2024-25. The question answers and explanations of ex. 3.3 of 8th Maths are revised according to new syllabus and revised NCERT books for 2024-25 exams.
Class 8 Mathematics Chapter 3 Exercise 3.3 Solution
Download the free PDF file of Class VIII Mathematics NCERT book, Exercise 3.3 of chapter 3 Understanding Quadrilaterals. All the solutions for the questions in exercise 3.3 of class 8 mathematics are presented in a step-by-step manner. Our CBSE solutions are provided in a simple and easy-to-understand way. If you encounter any issues while using the NCERT Solution App or website, feel free to contact us without any hesitation.
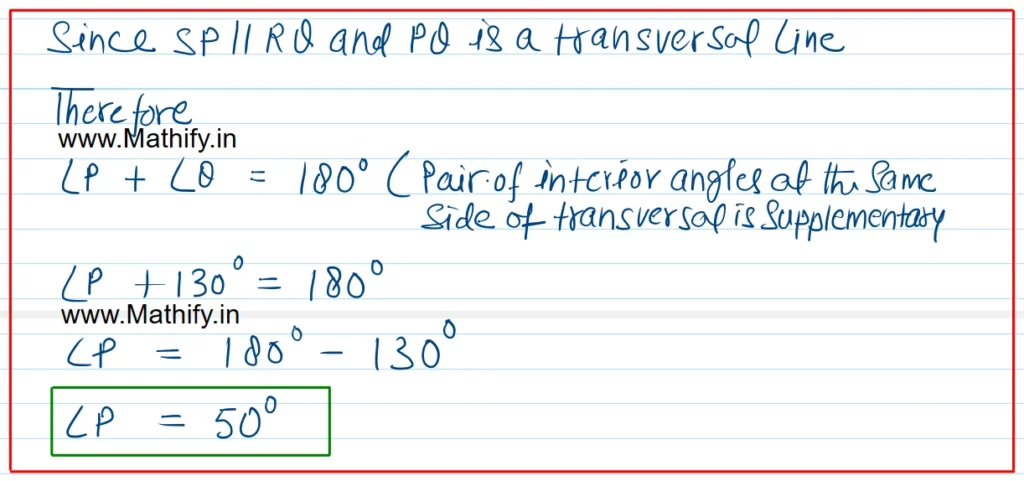
Q. 1. Given a parallelogram ABCD. Complete each statement along with the definition or property used.
(i) AD = _
(ii) ∠DCB = _
(iii) OC = _
(iv) m ∠DAB + ∠CDA = _

Solution:-
Solution:-
(i) AD = BC (Opposite side of a parallelogram are equal)
(ii) ∠DCB = ∠DCB (Opposite angles of a parallelogram are equal)
(iii) OC = AO = (Diagonals of a parallelogram bisects each other)
(iv) m∠DAB + m∠CDA = 180° (Adjacent angles in a parallelogram are supplementary)
Solution:-
Solution (i)-
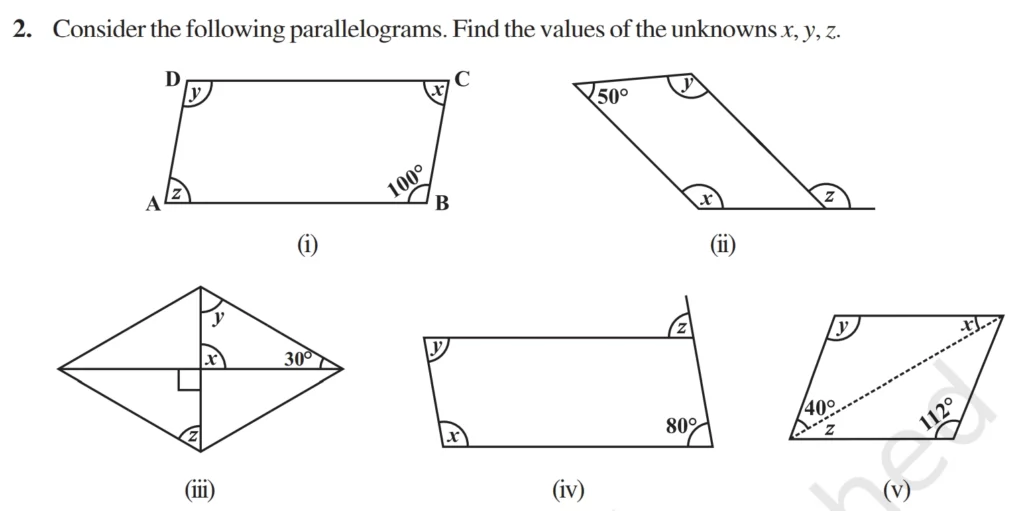
=≫ ∠y = ∠ABC = 100° (Opposite angles of a parallelograms are equal)
=≫ ∠x° + ∠100° = 180° (Adjacent angles in a parallelogram are supplementary)
=≫ ∠x° = 180° – 100°
=≫ ∠x° = 80°
=≫ ∠z = ∠x° = 80° (Opposite angles of a parallelograms are equal)
Solution (ii):-
=≫ ∠x° + ∠50° = 180° (Adjacent angles in a parallelogram are supplementary)
=≫ ∠x° = 180° – ∠50°
=≫ ∠x° = 130°
=≫ ∠x° = ∠y° = 130° (Opposite angles of a parallelograms are equal)
Let the opposite angle of ∠50° is ∠a
=≫ ∠a = ∠50° (Opposite angles of a parallelograms are equal)
=≫ ∠a + ∠z = 180° (linear Pair)
=≫ 50° + ∠z = 180°
=≫ ∠z = 180° – 50°
=≫ ∠z = 130°
Solution (iii):-
∠x° = 90° (vertically opposite angles are equal)
∠x° + ∠y + 30° = 180° (angle sum property of a triangle)
90° + ∠y° + 30° = 180°
∠y° = 180° – 90° – 30°
∠y° = 60°
∠y° = ∠z° = 60° (alternate interior angles are equal)
Solution (iv):-
∠y° = 80° (opposite angles of a parallelogram are equal)
∠x° + 80° = 180° (Adjacent angles in a parallelogram are supplementary)
∠x° = 180° – 80°
∠x° = 100°
Let the opposite angle of ∠x° be ∠a°
∠a° = ∠x° = 100°
∠a° + ∠z° = 180° (Linear Pair)
100° + ∠z° = 180°
∠z° = 180° – 100°
∠z° = 80°
Solution (v):-
∠y° = 112° (opposite angles of a parallelogram are equal)
∠x° + ∠y° + 40° = 180° (angle sum property of a triangle)
∠x° + ∠112° + 40° = 180°
∠x° = 180° – 112° – 40°
∠x° = 28°
∠z° = ∠x° = 28° (interior opposite angles are equal)
Final Answers
∠x° = 28°, ∠y° = 112°, ∠z° = 28°

Solution:-

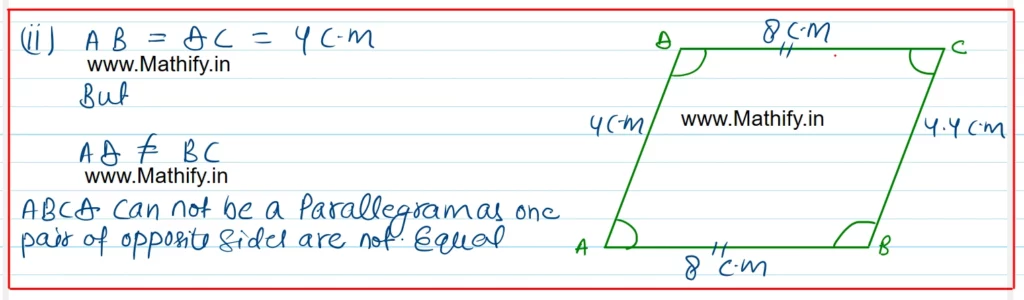
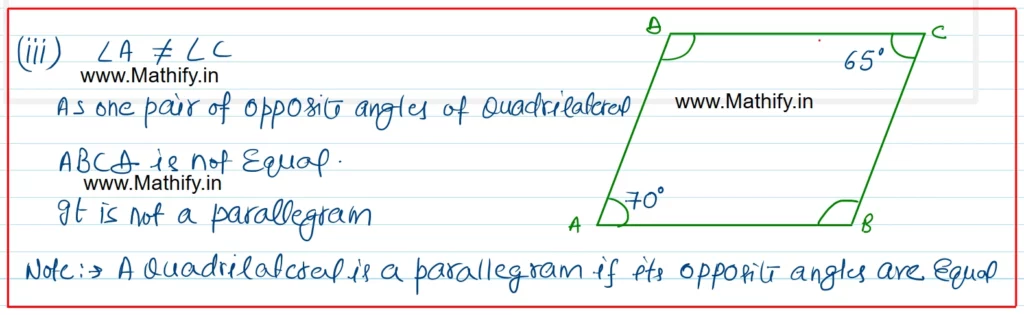

Solution:-
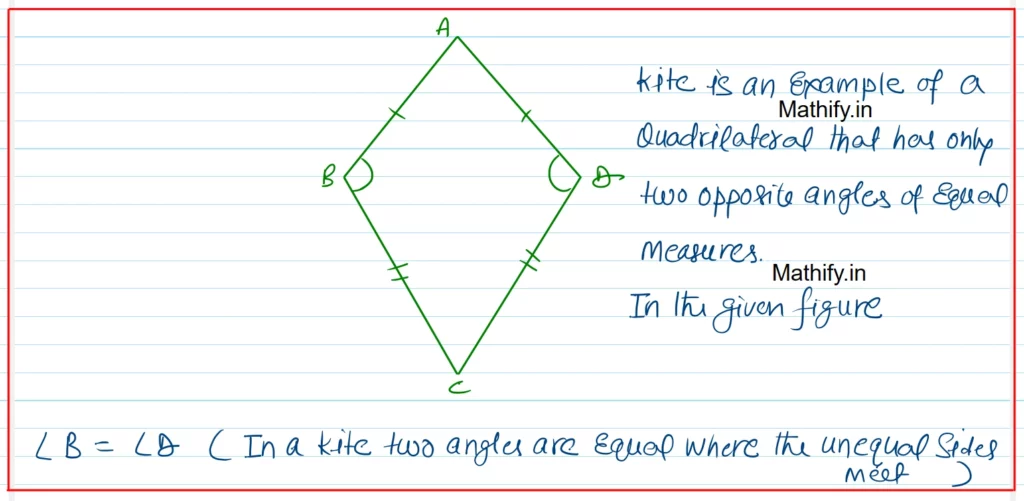

Solution:-
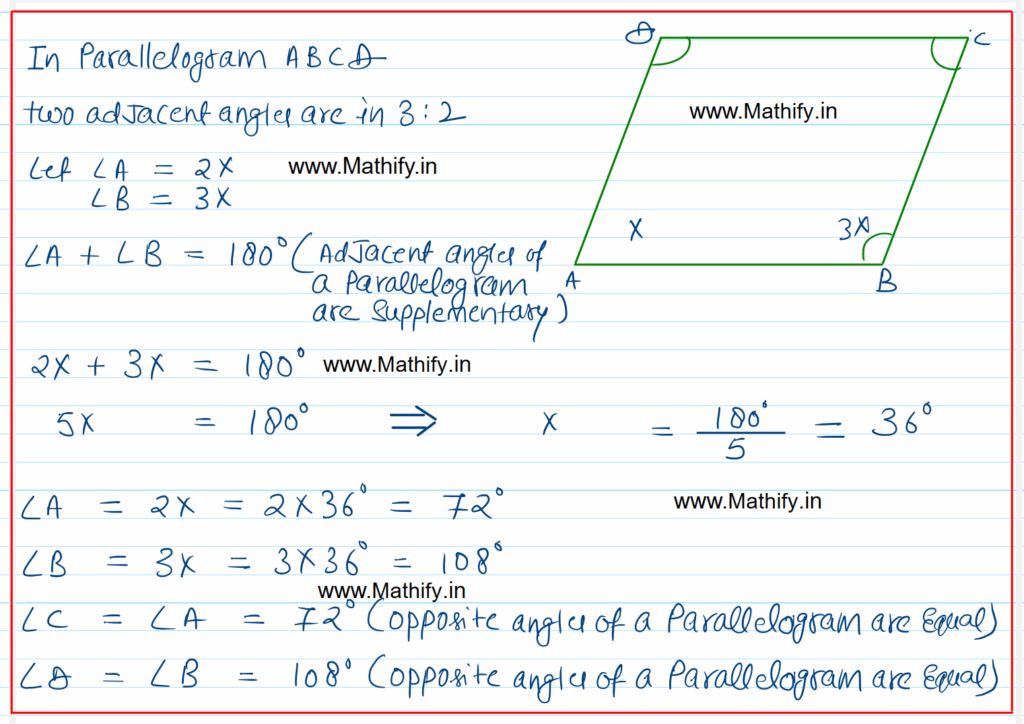

Solution:-
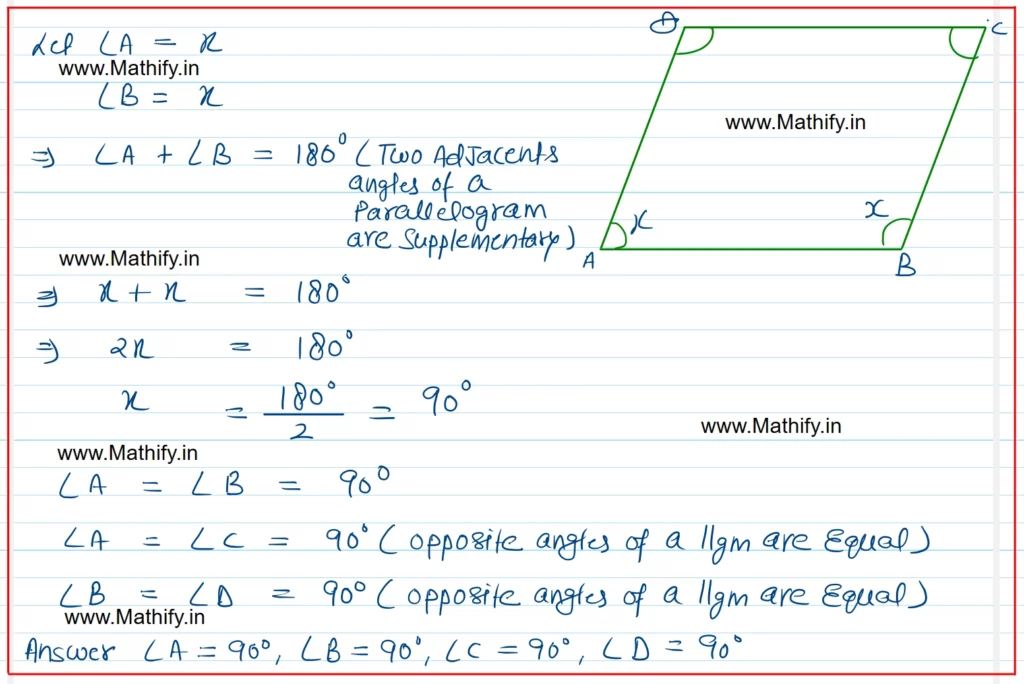

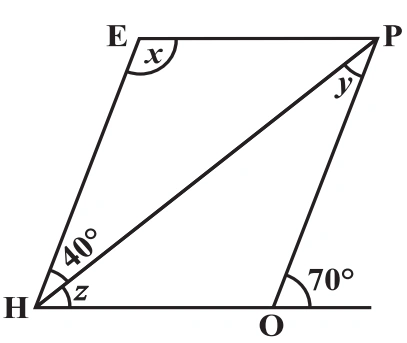
Solution:-
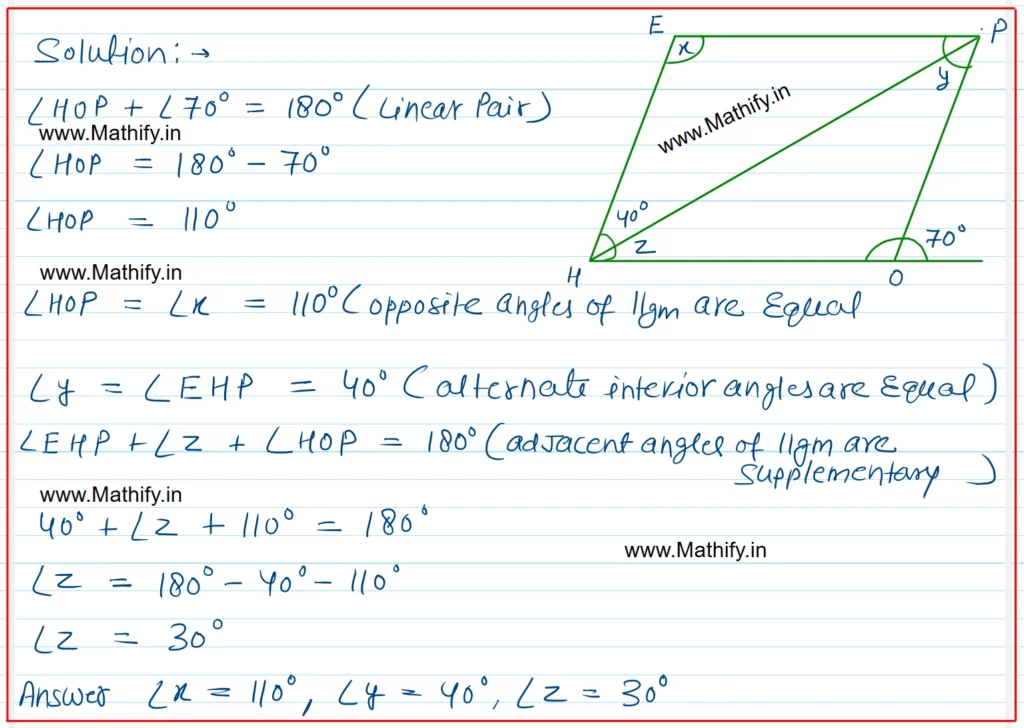

Solution:-

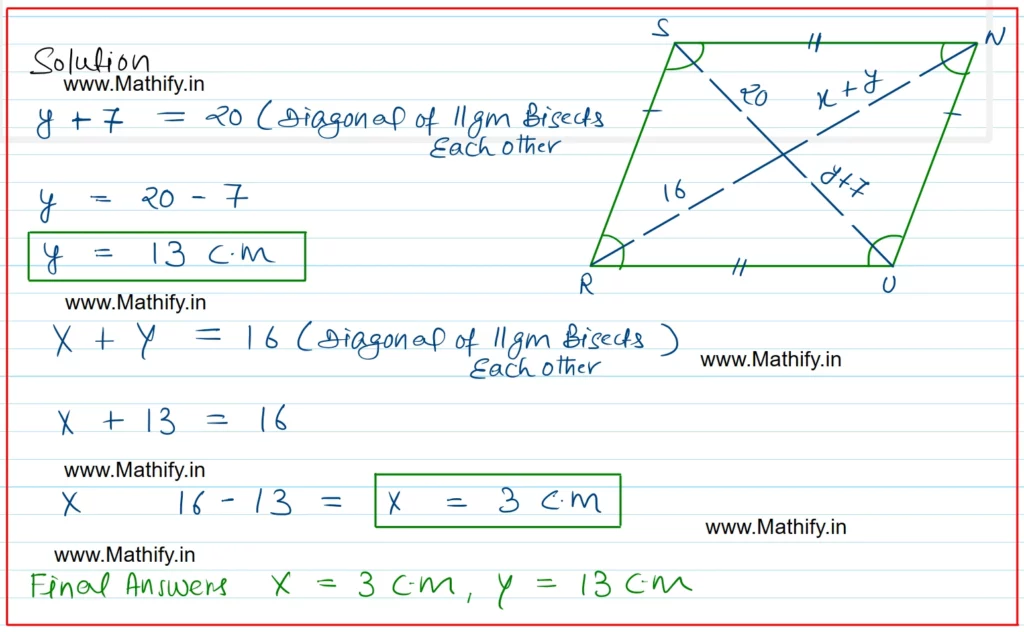
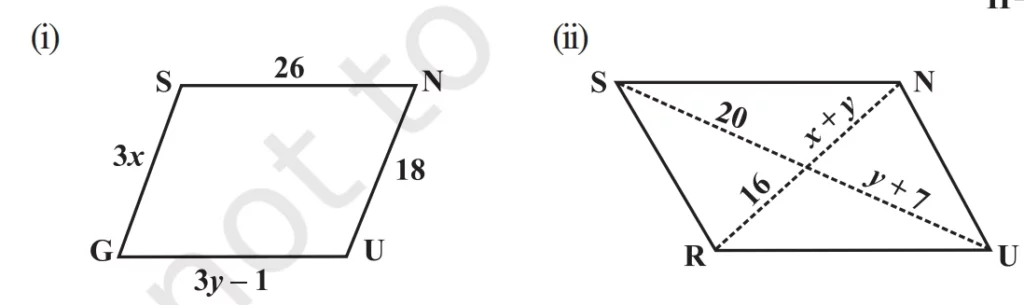
Solution:-
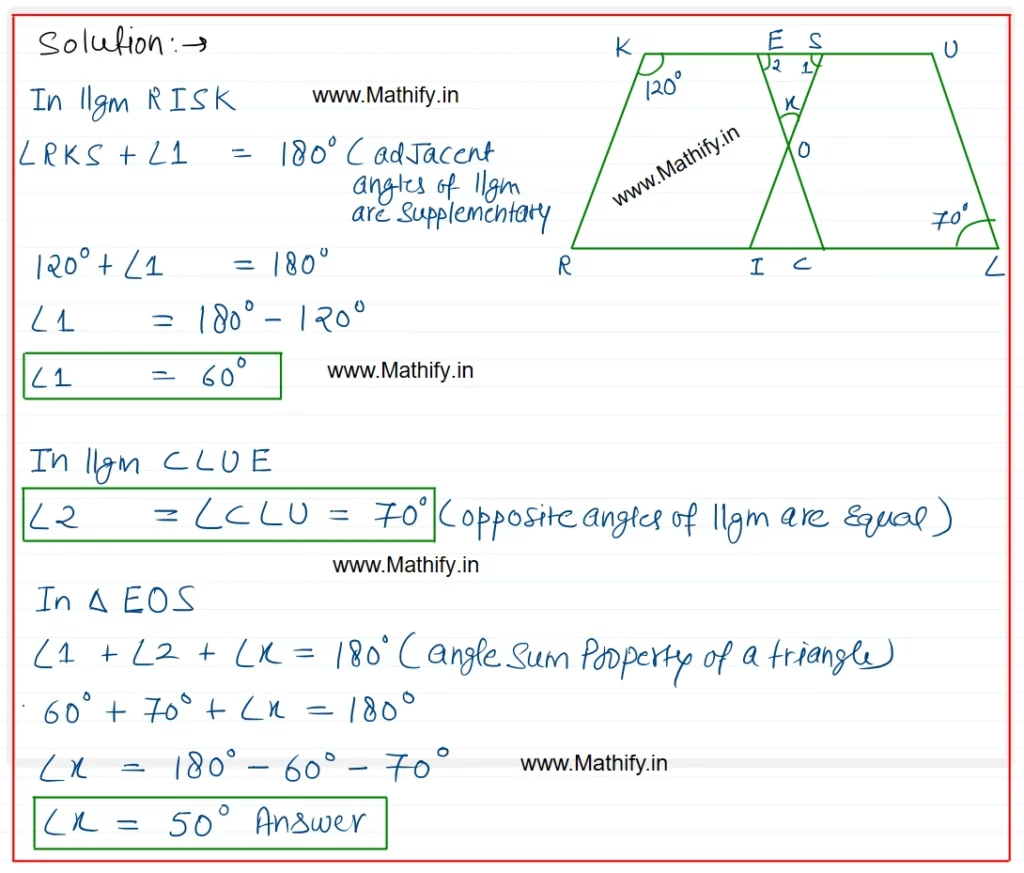

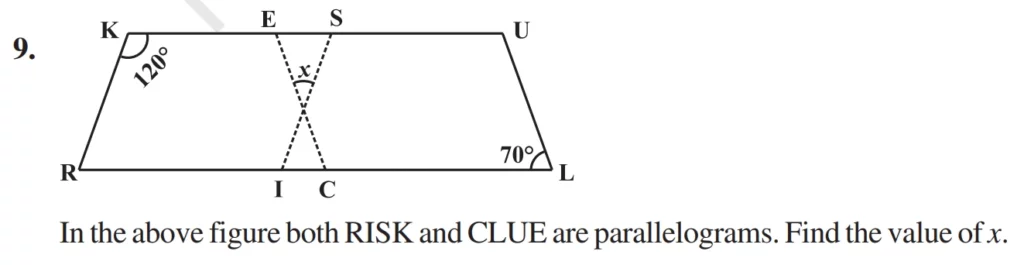
Solution:-

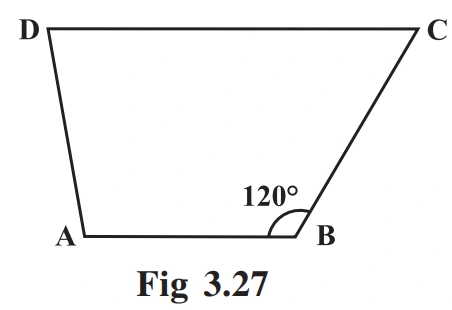
Solution:-


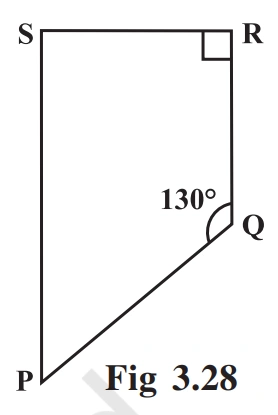
Solution:-